Monthly Archives: March 2024

My experimental adventures in quantum thermodynamics

Posted on by
Reply

Imagine a billiard ball bouncing around on a pool table. High-school level physics enables us to predict its motion until the end of time using simple equations for energy and momentum conservation, as long as you know the initial conditions – how fast the ball is moving at launch, and in which direction.

What if you add a second ball? This makes things more complicated, but predicting the future state of this system would still be possible based on the same principles. What about if you had a thousand balls, or a million? Technically, you could still apply the same equations, but the problem would not be tractable in any practical sense.

Billiard balls bouncing around on a pool table are a good analogy for a many-body system like a gas of molecules. Image credit

Thermodynamics lets us make precise predictions about averaged (over all the particles) properties of complicated, many-body systems, like millions of billiard balls or atoms bouncing around, without needing to know the gory details. We can make these predictions by introducing the notion of probabilities. Even though the system is deterministic – we can in principle calculate the exact motion of every ball – there are so many balls in this system, that the properties of the whole will be very close to the average properties of the balls. If you throw a six-sided die, the result is in principle deterministic and predictable, based on the way you throw it, but it’s in practice completely random to you – it could be 1 through 6, equally likely. But you know that if you cast a thousand dice, the average will be close to 3.5 – the average of all possibilities. Statistical physics enables us to calculate a probability distribution over the energies of the balls, which tells us everything about the average properties of the system. And because of entropy – the tendency for the system to go from ordered to disordered configurations, even if the probability distribution of the initial system is far from the one statistical physics predicts, after the system is allowed to bounce around and settle, this final distribution will be extremely close to a generic distribution that depends on average properties only. We call this the thermal distribution, and the process of the system mixing and settling to one of the most likely configurations – thermalization.

For a practical example – instead of billiard balls, consider a gas of air molecules bouncing around. The average energy of this gas is proportional to its temperature, which we can calculate from the probability distribution of energies. Being able to predict the temperature of a gas is useful for practical things like weather forecasting, cooling your home efficiently, or building an engine. The important properties of the initial state we needed to know – energy and number of particles – are conserved during the evolution, and we call them “thermodynamic charges”. They don’t actually need to be electric charges, although it is a good example of something that’s conserved.

Let’s cross from the classical world – balls bouncing around – to the quantum one, which deals with elementary particles that can be entangled, or in a superposition. What changes when we introduce this complexity? Do systems even thermalize in the quantum world? Because of the above differences, we cannot in principle be sure that the mixing and settling of the system will happen just like in the classical cases of balls or gas molecules colliding.

A visualization of a complex pattern called a quantum scar that can develop in quantum systems. Image credit

It turns out that we can predict the thermal state of a quantum system using very similar principles and equations that let us do this in the classical case. Well, with one exception – what if we cannot simultaneously measure our critical quantities – the charges?

One of the quirks of quantum mechanics is that observing the state of the system can change it. Before the observation, the system might be in a quantum superposition of many states. After the observation, a definite classical value will be recorded on our instrument – we say that the system has collapsed to this state, and thus changed its state. There are certain observables that are mutually incompatible – we cannot know their values simultaneously, because observing one definite value collapses the system to a state in which the other observable is in a superposition. We call these observables noncommuting, because the order of observation matters – unlike in multiplication of numbers, which is a commuting operation you’re familiar with. 2 * 3 = 6, and also 3 * 2 = 6 – the order of multiplication doesn’t matter.

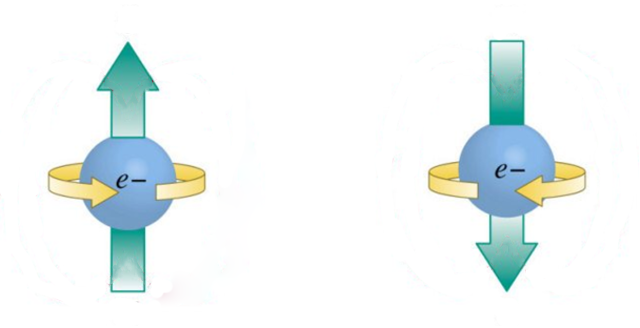
Electron spin is a common example that entails noncommutation. In a simplified picture, we can think of spin as an axis of rotation of our electron in 3D space. Note that the electron doesn’t actually rotate in space, but it is a useful analogy – the property is “spin” for a reason. We can measure the spin along the x-,y-, or z-axis of a 3D coordinate system and obtain a definite positive or negative value, but this observation will result in a complete loss of information about spin in the other two perpendicular directions.

An illustration of electron spin. We can imagine it as an axis in 3D space that points in a particular direction. Image from Wikimedia Commons.

If we investigate a system that conserves the three spin components independently, we will be in a situation where the three conserved charges do not commute. We call them “non-Abelian” charges, because they enjoy a non-Abelian, that is, noncommuting, algebra. Will such a system thermalize, and if so, to what kind of final state?

This is precisely what we set out to investigate. Noncommutation of charges breaks usual derivations of the thermal state, but researchers have managed to show that with non-Abelian charges, a subtly different non-Abelian thermal state (NATS) should emerge. Myself and Nicole Yunger Halpern at the Joint Center for Quantum Information and Computer Science (QuICS) at the University of Maryland have collaborated with Amir Kalev from the Information Sciences Institute (ISI) at the University of Southern California, and experimentalists from the University of Innsbruck (Florian Kranzl, Manoj Joshi, Rainer Blatt and Christian Roos) to observe thermalization in a non-Abelian system – and we’ve recently published this work in PRX Quantum .

The experimentalists used a device that can trap ions with electric fields, as well as manipulate and read out their states using lasers. Only select energy levels of these ions are used, which effectively makes them behave like electrons. The laser field can couple the ions in a way that approximates the Heisenberg Hamiltonian – an interaction that conserves the three total spin components individually. We thus construct the quantum system we want to study – multiple particles coupled with interactions that conserve noncommuting charges.

We conceptually divide the ions into a system of interest and an environment. The system of interest, which consists of two particles, is what we want to measure and compare to theoretical predictions. Meanwhile, the other ions act as the effective environment for our pair of ions – the environment ions interact with the pair in a way that simulates a large bath exchanging heat and spin.

Photo of our University of Maryland group. From left to right: Twesh Upadhyaya, Billy Braasch, Shayan Majidy, Nicole Yunger Halpern, Aleks Lasek, Jose Antonio Guzman, Anthony Munson.

If we start this total system in some initial state, and let it evolve under our engineered interaction for a long enough time, we can then measure the final state of the system of interest. To make the NATS distinguishable from the usual thermal state, I designed an initial state that is easy to prepare, and has the ions pointing in directions that result in high charge averages and relatively low temperature. High charge averages make the noncommuting nature of the charges more pronounced, and low temperature makes the state easy to distinguish from the thermal background. However, we also show that our experiment works for a variety of more-arbitrary states.

We let the system evolve from this initial state for as long as possible given experimental limitations, which was 15 ms. The experimentalists then used quantum state tomography to reconstruct the state of the system of interest. Quantum state tomography makes multiple measurements over many experimental runs to approximate the average quantum state of the system measured. We then check how close the measured state is to the NATS. We have found that it’s about as close as one can expect in this experiment!

And we know this because we have also implemented a different coupling scheme, one that doesn’t have non-Abelian charges. The expected thermal state in the latter case was reached within a distance that’s a little smaller than our non-Abelian case. This tells us that the NATS is almost reached in our experiment, and so it is a good, and the best known, thermal state for the non-Abelian system – we have compared it to competitor thermal states.

Working with the experimentalists directly has been a new experience for me. While I was focused on the theory and analyzing the tomography results they obtained, they needed to figure out practical ways to realize what we asked of them. I feel like each group has learned a lot about the tasks of the other. I have become well acquainted with the trapped ion experiment and its capabilities and limitation. Overall, it has been great collaborating with the Austrian group.

Our result is exciting, as it’s the first experimental observation within the field of non-Abelian thermodynamics! This result was observed in a realistic, non-fine-tuned system that experiences non-negligible errors due to noise. So the system does thermalize after all. We have also demonstrated that the trapped ion experiment of our Austrian friends can be used to simulate interesting many-body quantum systems. With different settings and programming, other types of couplings can be simulated in different types of experiments.

The experiment also opened avenues for future work. The distance to the NATS was greater than the analogous distance to the Abelian system. This suggests that thermalization is inhibited by the noncommutation of charges, but more evidence is needed to justify this claim. In fact, our other recent paper in Physical Review B suggests the opposite!

As noncommutation is one of the core features that distinguishes classical and quantum physics, it is of great interest to unravel the fine differences non-Abelian charges can cause. But we also hope that this research can have practical uses. If thermalization is disrupted by noncommutation of charges, engineered systems featuring them could possibly be used to build quantum memory that is more robust, or maybe even reduce noise in quantum computers. We continue to explore noncommutation, looking for interesting effects that we can pin on it. I am currently working on verifying the workings of a hypothesis that explains when and why quantum systems thermalize internally.

Noncommuting charges are much like Batman

Posted on by
1
The Noncommuting-Charges World Tour Part 2 of 4

This is the second part of a four-part series covering the recent Perspective on noncommuting charges. I’ll post one part every ~5 weeks leading up to my PhD thesis defence. You can find part 1 here.

Understanding a character’s origins enriches their narrative and motivates their actions. Take Batman as an example: without knowing his backstory, he appears merely as a billionaire who might achieve more by donating his wealth rather than masquerading as a bat to combat crime. However, with the context of his tragic past, Batman transforms into a symbol designed to instill fear in the hearts of criminals. Another example involves noncommuting charges. Without understanding their origins, the question “What happens when charges don’t commute?” might appear contrived or simply devised to occupy quantum information theorists and thermodynamicists. However, understanding the context of their emergence, we find that numerous established results unravel, for various reasons, in the face of noncommuting charges. In this light, noncommuting charges are much like Batman; their backstory adds to their intrigue and clarifies their motivation. Admittedly, noncommuting charges come with fewer costumes, outside the occasional steampunk top hat my advisor Nicole Yunger Halpern might sport.

Growing up, television was my constant companion. Of all the shows I’d get lost in, ‘Batman: The Animated Series’ stands the test of time. I highly recommend giving it a watch.

In the early works I’m about to discuss, a common thread emerges: the initial breakdown of some well-understood derivations and the effort to establish a new derivation that accommodates noncommuting charges. These findings will illuminate, yet not fully capture, the multitude of results predicated on the assumption that charges commute. Removing this assumption is akin to pulling a piece from a Jenga tower, triggering a cascade of other results. Critics might argue, “If you’re merely rederiving known results, this field seems uninteresting.” However, the reality is far more compelling. As researchers diligently worked to reconstruct this theoretical framework, they have continually uncovered ways in which noncommuting charges might pave the way for new physics. That said, the exploration of these novel phenomena will be the subject of my next post, where we delve into the emerging physics. So, I invite you to stay tuned. Back to the history…

E.T. Jaynes’s 1957 formalization of the maximum entropy principle has a blink-and-you’ll-miss-it reference to noncommuting charges. Consider a quantum system, similar to the box discussed in Part 1, where our understanding of the system’s state is limited to the expectation values of certain observables. Our aim is to deduce a probability distribution for the system’s potential pure states that accurately reflects our knowledge without making unjustified assumptions. According to the maximum entropy principle, this objective is met by maximizing the entropy of the distribution, which serve as a measure of uncertainty. This resulting state is known as the generalized Gibbs ensemble. Jaynes noted that this reasoning, based on information theory for the generalized Gibbs ensemble, remains valid even when our knowledge is restricted to the expectation values of noncommuting charges. However, later scholars have highlighted that physically substantiating the generalized Gibbs ensemble becomes significantly more challenging when the charges do not commute. Due to this and other reasons, when the system’s charges do not commute, the generalized Gibbs ensemble is specifically referred to as the non-Abelian thermal state (NATS).

For approximately 60 years, discussions about noncommuting charges remain dormant, outside a few mentions here and there. This changed when two studies highlighted how noncommuting charges break commonplace thermodynamics derivations. The first of these, conducted by Matteo Lostaglio as part of his 2014 thesis, challenged expectations about a system’s free energy—a measure of the system’s capacity for performing work. Interestingly, one can define a free energy for each charge within a system. Imagine a scenario where a system with commuting charges comes into contact with an environment that also has commuting charges. We then evolve the system such that the total charges in both the system and the environment are conserved. This evolution alters the system’s information content and its correlation with the environment. This change in information content depends on a sum of terms. Each term depends on the average change in one of the environment’s charges and the change in the system’s free energy for that same charge. However, this neat distinction of terms according to each charge breaks down when the system and environment exchange noncommuting charges. In such cases, the terms cannot be cleanly attributed to individual charges, and the conventional derivation falters.

The second work delved into resource theories, a topic discussed at length in Quantum Frontiers blog posts. In short, resource theories are frameworks used to quantify how effectively an agent can perform a task subject to some constraints. For example, consider all allowed evolutions (those conserving energy and other charges) one can perform on a closed system. From these evolutions, what system can you not extract any work from? The answer is systems in thermal equilibrium. The method used to determine the thermal state’s structure also fails when the system includes noncommuting charges. Building on this result, three groups (one, two, and three) presented physically motivated derivations of the form of the thermal state for systems with noncommuting charges using resource-theory-related arguments. Ultimately, the form of the NATS was recovered in each work.

Just as re-examining Batman’s origin story unveils a deeper, more compelling reason behind his crusade against crime, diving into the history and implications of noncommuting charges reveals their untapped potential for new physics. Behind every mask—or theory—there can lie an untold story. Earlier, I hinted at how reevaluating results with noncommuting charges opens the door to new physics. A specific example, initially veiled in Part 1, involves the violation of the Onsager coefficients’ derivation by noncommuting charges. By recalculating these coefficients for systems with noncommuting charges, we discover that their noncommutation can decrease entropy production. In Part 3, we’ll delve into other new physics that stems from charges’ noncommutation, exploring how noncommuting charges, akin to Batman, can really pack a punch.

The quantum gold rush

Posted on by
Reply

Even if you don’t recognize the name, you probably recognize the saguaro cactus. It’s the archetype of the cactus, a column from which protrude arms bent at right angles like elbows. As my husband pointed out, the cactus emoji is a saguaro: 🌵. In Tucson, Arizona, even the airport has a saguaro crop sufficient for staging a Western short film. I didn’t have a film to shoot, but the garden set the stage for another adventure: the ITAMP winter school on quantum thermodynamics.

Tucson airport

ITAMP is the Institute for Theoretical Atomic, Molecular, and Optical Physics (the Optical is silent). Harvard University and the Smithsonian Institute share ITAMP, where I worked as a postdoc. ITAMP hosted the first quantum-thermodynamics conference to take place on US soil, in 2017. Also, ITAMP hosts a winter school in Arizona every February. (If you lived in the Boston area, you might want to escape to the southwest then, too.) The winter school’s topic varies from year to year. 

How about a winter school on quantum thermodynamics? ITAMP’s director, Hossein Sadeghpour, asked me when I visited Cambridge, Massachusetts last spring.

Let’s do it, I said. 

Lecturers came from near and far. Kanu Sinha, of the University of Arizona, spoke about how electric charges fluctuate in the quantum vacuum. Fluctuations feature also in extensions of the second law of thermodynamics, which helps explain why time flows in only one direction. Gabriel Landi, from the University of Rochester, lectured about these fluctuation relations. ITAMP Postdoctoral Fellow Ceren Dag explained why many-particle quantum systems register time’s arrow. Ferdinand Schmidt-Kaler described the many-particle quantum systems—the trapped ions—in his lab at the University of Mainz.

Ronnie Kosloff, of Hebrew University in Jerusalem, lectured about quantum engines. Nelly Ng, an Assistant Professor at Nanyang Technological University, has featured on Quantum Frontiers at least three times. She described resource theories—information-theoretic models—for thermodynamics. Information and energy both serve as resources in thermodynamics and computation, I explained in my lectures.

The 2024 ITAMP winter school

The winter school took place at the conference center adjacent to Biosphere 2. Biosphere 2 is an enclosure that contains several miniature climate zones, including a coastal fog desert, a rainforest, and an ocean. You might have heard of Biosphere 2 due to two experiments staged there during the 1990s: in each experiment, a group of people was sealed in the enclosure. The experimentalists harvested their own food and weren’t supposed to receive any matter from outside. The first experiment lasted for two years. The group, though, ran out of oxygen, which a support crew pumped in. Research at Biosphere 2 contributes to our understanding of ecosystems and space colonization.

Fascinating as the landscape inside Biosphere 2 is, so is the landscape outside. The winter school included an afternoon hike, and my husband and I explored the territory around the enclosure.

Did you see any snakes? my best friend asked after I returned home.

No, I said. But we were chased by a vicious beast. 

On our first afternoon, my husband and I followed an overgrown path away from the biosphere to an almost deserted-looking cluster of buildings. We eventually encountered what looked like a warehouse from which noises were emanating. Outside hung a sign with which I resonated.

Scientists, I thought. Indeed, a researcher emerged from the warehouse and described his work to us. His group was preparing to seal off a building where they were simulating a Martian environment. He also warned us about the territory we were about to enter, especially the creature that roosted there. We were too curious to retreat, though, so we set off into a ghost town.

At least, that’s what the other winter-school participants called the area, later in the week—a ghost town. My husband and I had already surveyed the administrative offices, conference center, and other buildings used by biosphere personnel today. Personnel in the 1980s used a different set of buildings. I don’t know why one site gave way to the other. But the old buildings survive—as what passes for ancient ruins to many Americans. 

Weeds have grown up in the cracks in an old parking lot’s tarmac. A sign outside one door says, “Classroom”; below it is a sign that must not have been correct in decades: “Class in progress.” Through the glass doors of the old visitors’ center, we glimpsed cushioned benches and what appeared to be a diorama exhibit; outside, feathers and bird droppings covered the ground. I searched for a tumbleweed emoji, to illustrate the atmosphere, but found only a tumbler one: 🥃.

After exploring, my husband and I rested in the shade of an empty building, drank some of the water we’d brought, and turned around. We began retracing our steps past the defunct visitors’ center. Suddenly, a monstrous Presence loomed on our right. 

I can’t tell you how large it was; I only glimpsed it before turning and firmly not running away. But the Presence loomed. And it confirmed what I’d guessed upon finding the feathers and droppings earlier: the old visitors’ center now served as the Lair of the Beast.

The Mars researcher had warned us about the aggressive male turkey who ruled the ghost town. The turkey, the researcher had said, hated men—especially men wearing blue. My husband, naturally, was wearing a blue shirt. You might be able to outrun him, the researcher added pensively.

My husband zipped up his black jacket over the blue shirt. I advised him to walk confidently and not too quickly. Hikes in bear country, as well as summers at Busch Gardens Zoo Camp, gave me the impression that we mustn’t run; the turkey would probably chase us, get riled up, and excite himself to violence. So we walked, and the monstrous turkey escorted us. For surprisingly and frighteningly many minutes. 

The turkey kept scolding us in monosyllabic squawks, which sounded increasingly close to the back of my head. I didn’t turn around to look, but he sounded inches away. I occasionally responded in the soothing voice I was taught to use on horses. But my husband and I marched increasingly quickly.

We left the old visitors’ center, curved around, and climbed most of a hill before ceasing to threaten the turkey—or before he ceased to threaten us. He squawked a final warning and fell back. My husband and I found ourselves amid the guest houses of workshops past, shaky but unmolested. Not that the turkey wreaks much violence, according to the Mars researcher: at most, he beats his wings against people and scratches up their cars (especially blue ones). But we were relieved to return to civilization.

Afternoon hike at Catalina State Park, a drive away from Biosphere 2. (Yes, that’s a KITP hat.)

The ITAMP winter school reminded me of Roughing It, a Mark Twain book I finished this year. Twain chronicled the adventures he’d experienced out West during the 1860s. The Gold Rush, he wrote, attracted the top young men of all nations. The quantum-technologies gold rush has been attracting the top young people of all nations, and the winter school evidenced their eagerness. Yet the winter school also evidenced how many women have risen to the top: 10 of the 24 registrants were women, as were four of the seven lecturers.1 

The winter-school participants in the shuttle I rode from the Tucson airport to Biosphere 2

We’ll see to what extent the quantum-technologies gold rush plays out like Mark Twain’s. Ours at least involves a ghost town and ferocious southwestern critters.

1For reference, when I applied to graduate programs, I was told that approximately 20% of physics PhD students nationwide were women. The percentage of women drops as one progresses up the academic chain to postdocs and then to faculty members. And primarily PhD students and postdocs registered for the winter school.