May | 2017 | Quantum Frontiers

Sara Imari Walker studies ants. Her entomologist colleague Gabriele Valentini cultivates ant swarms. Gabriele coaxes a swarm from its nest, hides the nest, and offers two alternative nests. Gabriele observe the ants’ responses, then analyzes their data with Sara.

Sara doesn’t usually study ants. She trained in physics, information theory, and astrobiology. (Astrobiology is the study of life; life’s origins; and conditions amenable to life, on Earth and anywhere else life may exist.) Sara analyzes how information reaches, propagates through, and manifests in the swarm.

Some ants inspect one nest; some, the other. Few ants encounter both choices. Yet most of the ants choose simultaneously. (How does Gabriele know when an ant chooses? Decided ants carry other ants toward the chosen nest. Undecided ants don’t.)

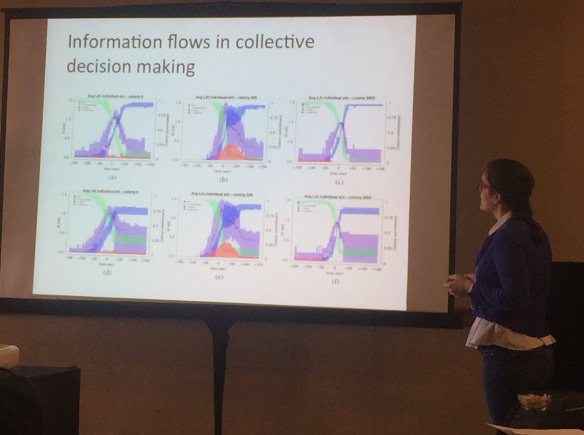
Gabriele and Sara plotted each ant’s status (decided or undecided) at each instant. All the ants’ lines start in the “undecided” region, high up in the graph. Most lines drop to the “decided” region together. Physicists call such dramatic, large-scale changes in many-particle systems “phase transitions.” The swarm transitions from the “undecided” phase to the “decided,” as moisture transitions from vapor to downpour.

Sara versus the ants

Look from afar, and you’ll see evidence of a hive mind: The lines clump and slump together. Look more closely, and you’ll find lags between ants’ decisions. Gabriele and Sara grouped the ants according to their behaviors. Sara explained the grouping at a workshop this spring.

The green lines, she said, are undecided ants.

My stomach dropped like Gabriele and Sara’s ant lines.

People call data “cold” and “hard.” Critics lambast scientists for not appealing to emotions. Politicians weave anecdotes into their numbers, to convince audiences to care.

But when Sara spoke, I looked at her green lines and thought, “That’s me.”

I’ve blogged about my indecisiveness. Postdoc Ning Bao and I formulated a quantum voting scheme in which voters can superpose—form quantum combinations of—options. Usually, when John Preskill polls our research group, I abstain from voting. Politics, and questions like “Does building a quantum computer require only engineering or also science?”,¹ have many facets. I want to view such questions from many angles, to pace around the questions as around a sculpture, to hear other onlookers, to test my impressions on them, and to cogitate before choosing.² However many perspectives I’ve gathered, I’m missing others worth seeing. I commiserated with the green-line ants.

I first met Sara in the building behind the statue. Sara earned her PhD in Dartmouth College’s physics department, with Professor Marcelo Gleiser.

Sara presented about ants at a workshop hosted by the Beyond Center for Fundamental Concepts in Science at Arizona State University (ASU). The organizers, Paul Davies of Beyond and Andrew Briggs of Oxford, entitled the workshop “The Power of Information.” Participants represented information theory, thermodynamics and statistical mechanics, biology, and philosophy.

Paul and Andrew posed questions to guide us: What status does information have? Is information “a real thing” “out there in the world”? Or is information only a mental construct? What roles can information play in causation?

We paced around these questions as around a Chinese viewing stone. We sat on a bench in front of those questions, stared, debated, and cogitated. We taught each other about ants, artificial atoms, nanoscale machines, and models for information processing.

Chinese viewing stone in Yuyuan Garden in Shanghai

I wonder if I’ll acquire opinions about Paul and Andrew’s questions. Maybe I’ll meander from “undecided” to “decided” over a career. Maybe I’ll phase-transition like Sara’s ants. Maybe I’ll remain near the top of her diagram, a green holdout.

I know little about information’s power. But Sara’s plot revealed one power of information: Information can move us—from homeless to belonging, from ambivalent to decided, from a plot’s top to its bottom, from passive listener to finding yourself in a green curve.

With thanks to Sara Imari Walker, Paul Davies, Andrew Briggs, Katherine Smith, and the Beyond Center for their hospitality and thoughts.

¹By “only engineering,” I mean not “merely engineering” pejoratively, but “engineering and no other discipline.”

²I feel compelled to perform these activities before choosing. I try to. Psychological experiments, however, suggest that I might decide before realizing that I’ve decided.

Being the physics department executive officer (on top of being a quantum physicist) makes me think a lot about our physics college program. It is exciting. We start with mechanics, and then go to electromagnetism (E&M) and relativity, then to quantum and statistical mechanics, and then to advanced mathematical methods, analytical mechanics and more E&M. The dessert is usually field theory, astrophysics and advanced lab. You can take some advanced courses, introducing condensed matter, quantum computation, particle theory, AMO, general relativity, nuclear physics, etc. By the time we are done with college, we definitely feel like we know a lot.

But in the end of all that, what do we know about modern physics? Certainly we all took a class called ‘modern physics’. Or should I say ‘”modern” physics’? Because, I’m guessing, the modern physics class heavily featured the Stern-Gerlach experiment (1922) and mentions of De-Broglie, Bohr, and Dirac quite often. Don’t get me wrong: great physics, and essential. But modern?

So what would be modern physics? What should we teach that does not predate 1960? By far the biggest development in my neck of the woods is easy access to computing power. Even I can run simulations for a Schroedinger equation (SE) with hundreds of sites and constantly driven. Even I can diagonalize a gigantic matrix that corresponds to a Mott-Hubbard model of 15 or maybe even 20 particles. What’s more, new approximate algorithms capture the many-body quantum dynamics, and ground states of chains with 100s of sites. These are the DMRG (density matrix renormalization group) and MPS (matrix product states) (see https://arxiv.org/abs/cond-mat/0409292 for a review of DMRG, and https://arxiv.org/pdf/1008.3477.pdf for a review of MPS, both by the inspiring Uli Schollwoeck).

Should we teach that? Isn’t it complicated? Yes and no. Respectively – not simultaneously. We should absolutely teach it. And no – it is really not complicated. That’s the point – it is simpler than Schroedinger’s equation! How do we teach it? I am not sure yet, but certainly there is a junior level time slot for computational quantum mechanics somewhere.

What else? Once we think about it, the flood gates open. Condensed matter just gave us a whole new paradigm for semi-conductors: topological insulators. Definitely need to teach that – and it is pure 21st century! Tough? Not at all, just solving SE on a lattice. Not tough? Well, maybe not trivial, but is it any tougher than finding the orbitals of Hydrogen? (at the risk of giving you nightmares, remember Laguerre polynomials? Oh – right – you won’t get any nightmares, because, most likely, you don’t remember!)

With that let me take a shot at the standard way that quantum mechanics is taught. Roughly a quantum class goes like this: wave-matter duality; SE; free particle; box; harmonic oscillator, spin, angular momentum, hydrogen atom. This is a good program for atomic physics, and possibly field theory. But by and large, this is the quantum mechanics of vacuum. What about quantum mechanics of matter? Is Feynman path integral really more important than electron waves in solids? All physics is beautiful. But can’t Feynman wait while we teach tight binding models?

And I’ll stop here, before I get started on hand-on labs, as well as the fragmented nature of our programs.

Question to you all out there: Suppose we go and modernize (no quotes) our physics program. What should we add? What should we take away? And we all agree – all physics is Beautiful! I’m sure I have my blind spots, so please comment!