Generations of kids had their first encounter with the explanation to the question: What does matter consist of? Atoms -> protons -> quarks. There is a certain pleasure in explaining this, regardless of your physics background. Just like a soldier polishes every new medal he gets, the story about elementary particles gets more exciting with every new ‘magic number’ introduced. Indeed, why 3 colors for quarks? A lay person would think that the inspiration came from the RGB (red-green-blue) colors in a TV tube. However, what physicists really did was try to say a new word in the language of gauge theories. And colored quarks was one of the first words one can say when learning to speak such a language: U(1), SU(2), SU(3)! The very third word.
So why don’t we try to share a scientific excitement about gauge theories?
Everybody studied electricity at school. Teachers liked to talk about elementary charges, flowing back and forth. It’s okay to postulate existence of an indivisible charge among other physical laws, but wouldn’t it be cool to explain it instead? So that this integral property would be intimately related with another postulated ability of the charge – to sense electromagnetic fields. And indeed, in gauge field theory of electromagnetism the only things that can sense our gauge electromagnetic field are representations of the corresponding gauge group U(1). Conveniently, they are labeled by integer numbers – the charge values.
What does it mean for a physical model to have a gauge group? Let’s start with a simpler case of having a symmetry group. For example, a model of a glass of water has z-axis rotation symmetry (also the U(1) group, by coincidence). Does the same glass of water have a gauge group?
In a sense, yes. We know that pessimists call it ‘half-empty’, while optimists – ‘half-filled’. Both descriptions refer to the same physical object, and are used by different people for different purposes. We have redundancy in our language when we talk about glasses of water, and to remove it we will have to choose between depression and bliss. Unless we make such choice, we have global gauge symmetry.
REMARK: If you want real science, read my note on hamiltonians with a gauge symmetry in the context of quantum information.

I love your remark that the half-empty/half-full dichotomy is a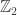 gauge symmetry. Sadly, I can’t follow the link to the google document for more details.
gauge symmetry. Sadly, I can’t follow the link to the google document for more details.
Thanks for pointing out the broken link Steve. It is now fixed.
This is the first explanation of gauge symmetry that I can understand. Thank you! Although I have no idea what you mean by “z-axis rotation.”