One line proof

Posted on by

It is not often that we come across a problem whose solution can fit in the margins of a notebook. In fact, many of the problems I have worked on in the field of quantum many-body physics require proofs that often exceed 30 pages. And that is without taking into account the several references included as sources for results used as “elementary” tools in the proof (referees love these papers…) So it is natural to think that a proof is a proof, so long as it is correct, and once confirmed by the academic community it is time to move on to something new.

But… sometimes things get interesting. A 30-page proof collapses to a 3-page proof when a different point of view is adopted (see the famous Prime Number Theorem). Below, you will find two problems that may, or may not have a “one-line” proof. The challenge is for you to find the shortest, most elegant proof for each problem:

A unit triangle: A triangle with sides a\le b \le c has area 1. Show that b^2 \ge 2.

Fermat’s Lost Theorem: Show that (x+y)^n = x^m+y^m has only one solution with positive integers x > y > 0 and m,n > 1.

In case you figure out the above problems (who knows, you guys are pretty good), you can try your luck with this one:

Magic Square Cubed: Show that there is no 3\times3 array of distinct cubed numbers (e.g. 8,27,125,\ldots) such that the sums of numbers in each row, each column and each of the two diagonals are all equal. That is, show that there is no magic square of cubes.

And if you are an alien supergenius, you can go for all the money:

Magic Square Superpower Challenge: Show that there is no 3\times3 array of n-th powers of distinct numbers with n\ge 4, such that the sums of numbers in each row, each column and each of the two diagonals are all equal. That is, show that there is no magic square of n-th powers, for n \ge 4.

The most elegant solution to the Superpower Challenge will receive a $100 prize. And in case you can show that there is no magic square of distinct squares… Nah, that’s impossible.

P.S.: To claim the prize, you and I should be able to understand your solution.

This entry was posted in The expert's corner by spiros. Bookmark the permalink.

About spiros

Spyridon Michalakis does research on quantum many-body physics and topological quantum order at Caltech's Institute for Quantum Information and Matter, where he is also the manager for outreach activities.

19 thoughts on “One line proof”

  1. A unit triangle: In a triangle a,b,c there is at most one angle >=90°. Let x,y be the two other angles <=90°, thus sin(alpha)<=1 and sin(beta)<=1. These two angles will be attached to the longest side c. Given two angles alpha, beta and the one side b (opposite of beta), we know the area A of a triangle is A=b² sin(alpha)sin(alpha+beta)/[2 sin(beta)]=1. Thus b²=2 sin(beta)/[sin(alpha)(sin(alpha+beta))]. But now sin(alpha)sin(alpha+beta)<=sin(alpha+beta)<=sin(beta), considering alpha,beta=1, therefore also b²>=2.

    Reply ↓

  4. The Unit Triangle answers above are the shortest, most elegant ones I can possibly imagine – one line proofs! Now on to Fermat’s Lost Theorem. Any geniuses willing to take a shot at it?

    Reply ↓

    • To start with, the single solution is
      (2+1)^2 = 2^3 + 1^3
      because both sides are equal to nine. Unfortunately there’s not enough room on this line to prove that there are no other solutions. 🙂

      Reply ↓

  5. Magic square cubed.

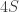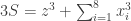    If S is the sum – of any row, column, or diagonal – then the sum of all 4 sums of lines going through the middle is 4S but it is also equal to 4z^3+\sum_{i=1}^8 x_i^3 where z^3 is the entry at the central square and x_i^3 are the remaining eight entries. The sum of all entries is also equal to 3S = z^3+\sum_{i=1}^8 x_i^3. The difference implies 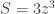S = 3z^3; the central entry is always the “average” of the entries. 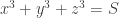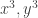Then x^3+y^3+z^3=S implies that x^3+y^3=2z^3 where x^3,y^3 are the opposing entries on a line going through the middle.

    I read that x^3+y^3=2z^3 has no solutions with distinct positive integers, as proved by Euler and Legendre, but I am not able to reproduce the proof now although it shouldn’t be too hard.

    The paid challenge has simply third powers replaced by higher powers in the text above. I think it is an extremely hard job for $100 as the sources indicate that one needs to prove the higher-exponent counterpart of the previous paragraph but the known ways to do so rely on Wiles’ proof of Fermat’s Last Theorem. 😉

    Reply ↓

  6. I am going to take a long shot here with the question regarding Fermat.

    Let’s take an ellipse here:
    (x/a)^2 + (y/b)^2 = 1 would define an ellipse.
    Let x/a = g, y/b = h
    Thus, (g + h)^m = g^2 + h^2 = 1 and that is the only possible solution with m,n> 1 and g> h> 0

    If g and h are ellipse’s major and minor axis respectively..

    I might be a bit confused here, too.

    Reply ↓

    • Dear Hiccup (cool name), I am about to post a partial solution to Fermat’s Lost Theorem. I hope you find it interesting and take the time to figure out the remaining part of the proof! Your questions will be answered within the upcoming post.

      Reply ↓

  7. Pingback: Fermat’s Lost Theorem | Quantum Frontiers

Your thoughts here.