Perhaps because my 40th wedding anniversary is just a few weeks away, I have been thinking about anniversaries lately, which reminded me that we are celebrating the 20th anniversary of a number of milestones in quantum information science. In 1995 Cirac and Zoller proposed, and Wineland’s group first demonstrated, the ion trap quantum computer. Quantum error-correcting codes were invented by Shor and Steane, entanglement concentration and purification were described by Bennett et al., and there were many other fast-breaking developments. It was an exciting year.
But the event that moved me to write a blog post is the 1995 appearance of the word “qubit” in an American Physical Society journal. When I was a boy, two-level quantum systems were called “two-level quantum systems.” Which is a descriptive name, but a mouthful and far from euphonious. Think of all the time I’ve saved in the past 20 years by saying “qubit” instead of “two-level quantum system.” And saying “qubit” not only saves time, it also conveys the powerful insight that a quantum state encodes a novel type of information. (Alas, the spelling was bound to stir controversy, with the estimable David Mermin a passionate holdout for “qbit”. Give it up, David, you lost.)
Ben Schumacher. Thanks for the qubits, Ben!
For the word “qubit” we know whom to thank: Ben Schumacher. He introduced the word in his paper “Quantum Coding” which appeared in the April 1995 issue of Physical Review A. (History is complicated, and in this case the paper was actually submitted in 1993, which allowed another paper by Jozsa and Schumacher to be published earlier even though it was written and submitted later. But I’m celebrating the 20th anniversary of the qubit now, because otherwise how will I justify this blog post?)
In the acknowledgments of the paper, Ben provided some helpful background on the origin of the word:
The term “qubit” was coined in jest during one of the author’s many intriguing and valuable conversations with W. K. Wootters, and became the initial impetus for this work.
I met Ben (and other luminaries of quantum information theory) for the first time at a summer school in Torino, Italy in 1996. After reading his papers my expectations were high, all the more so after Sam Braunstein warned me that I would be impressed: “Ben’s a good talker,” Sam assured me. I was not disappointed.
(I also met Asher Peres at that Torino meeting. When I introduced myself Asher asked, “Isn’t there someone with a similar name in particle theory?” I had no choice but to come clean. I particularly remember that conversation because Asher told me his secret motivation for studying quantum entanglement: it might be important in quantum gravity!)
A few years later Ben spent his sabbatical year at Caltech, which gave me an opportunity to compose a poem for the introduction to Ben’s (characteristically brilliant) talk at our physics colloquium. This poem does homage to that famous 1995 paper in which Ben not only introduced the word “qubit” but also explained how to compress a quantum state to the minimal number of qubits from which the original state can be recovered with a negligible loss of fidelity, thus formulating and proving the quantum version of Shannon’s famous source coding theorem, and laying the foundation for many subsequent developments in quantum information theory.
Sometimes when I recite a poem I can sense the audience’s appreciation. But in this case there were only a few nervous titters. I was going for edgy but might have crossed the line into bizarre.. Since then I’ve (usually) tried to be more careful.
(For reading the poem, it helps to know that the quantum state appears to be random when it has been compressed as much as possible.)
On Quantum Compression (in honor of Ben Schumacher)
Ben.
He rocks.
I remember
When
He showed me how to fit
A qubit
In a small box.I wonder how it feels
To be compressed.
And then to pass
A fidelity test.Or does it feel
At all, and if it does
Would I squeal
Or be just as I was?If not undone
I’d become as I’d begun
And write a memorandum
On being random.
Had it felt like a belt
Of rum?And might it be predicted
That I’d become addicted,
Longing for my session
Of compression?I’d crawl
To Ben again.
And call,
“Put down your pen!
Don’t stall!
Make me small!”
Quantum Frontiers 
Pingback: Who named the qubit? | Φυσ&io...
Pingback: Ποιος εισήγαγε πρώτος τον όρο qubit; | physicsgg
John, your poem is fantastic. One of these days I’m going to post my collection of your after-colloquium puns.
Thanks, Steve! Just the funny puns, I hope …
Some years ago, I asked Benjamin Schumacher, among a lot of other … celebrities ,,, in quanta, the following question, to which I have NOT yet gotten any answer from ANY of them : 🙂 🙂 🙂
Asher Peres, considered to be one of the fathers of quantum information, showed in his often cited 1995 book on quanta that one can build the whole of quantum theory WITHOUT the use of the Heisenberg uncertainty …
Details can be found in :
“Heisenberg’s Uncertainty : an Ill-Defined Notion ?”
http://hal.archives-ouvertes.fr/hal-00684501, http://vixra.org/abs/1203.0095
Amusingly, no matter how often that book is cited, it appears that NO ONE has bothered to look even at its … back cover … where in pretty large letters it is written that the Heisenberg Uncertainty is an … ill-defined notion … 🙂 🙂 🙂
Well, ever since, neither Schumacher, nor any other … quantum specialist … managed to give even the vaguest explanation of WHAT may be going on … 🙂 🙂 🙂
One more example of the fact that, well, quanta are NOT for … everybody …
Dear Prof. Rosinger,
On page 426 of Peres’ book, Heisenberg’s uncertainty principle is explicitly used to illustrate that classical reality emerges when our observations have uncertainties far beyond the quantum limit given by Heisenberg. Moreover, the uncertainty principle itself is *not needed* for quantum physics. It is a mathematical theorem about a lower bound in the product of the standard deviations of expectation values of complementary observables (an observable and its Fourier transform)[see Exercise 11.30 here: https://www.math.ucdavis.edu/~hunter/book/ch11.pdf ]. This theorem holds for all L_2 spaces. Quantum mechanics is formulated on such spaces, hence the uncertainty principle is a *consequence*, not a prerequisite, of the internal structure of these mathematical spaces. If you were to ask why quantum mechanics is formulated on L_2 spaces, that is a very interesting question whose answer may involve Dvoretzky’s theorem (random, low dimensional subspaces of high-dimensional L_p -normed spaces look a lot like L_2 spaces). Peres himself spends some time discussing the idea that macroscopic reality looks classical only because we can keep track of (measure) a tiny number of (macroscopic) degrees of freedom, say 10, in a system with 10^30 degrees of freedom. In Peres’ own view, that dimensional reduction generates classical ‘pointer states’ which are stable to quantum fluctuations and can be measured repeatedly by independent observers because of huge redundancy in the information they encode in their nearly identical subsystems (see also work on “Quantum Darwinism”, by W. H. Zurek). As Peres himself notes on p. 426 of “Quantum Theory: Concepts and Methods”: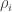
(our lack of knowledge is represented by an appropriately weighted mixture of all admissible possibilities). The accessible data usually are macroscopic variables, such as the position and velocity of the center of mass of an object. For these macroscopic variables, we have 
. Compared to these classical uncertainties, disturbances caused by quantum measurements are negligibly small. Moreover, macroscopic masses are large so that corresponding wavelengths are small and diffraction effects can be neglected. Therefore consecutive measurements of position have predictable results.”
“We can perform only a negligible fraction of all conceivable tests, and we therefore end up with a partial knowledge of
I hope this answers your long standing question.
Thank you for your effort in the detailed reply.
However, it does not touch in any way upon the MAIN issue, namely that Asher Peres calls in his 1995 book the Heisenberg Uncertainty to be an … ill-conceived notion … 🙂 🙂 🙂
I suppose, some points about not very accurate treatment of uncertainty relation are discussed in chapter 4-3 of Peres’ book, e.g., see Exercise 4.16 on page 94 (“Find three textbooks on quantum mechanics with the wrong uncertainty relation (4.56), and the one with correct version”) and text before that. Yet 4.56 is not usual uncertainty relation, it is for angular variable.
Sorry, you, too, AVOID the MAIN issue : Asher Peres calls the Heisenberg Uncertainty to be an … ill-conceived notion …
So then, why do you NOT comment on that ??? 🙂 🙂 🙂
Why his suggestion to find three textbooks with a wrong kind of a uncertainty relation is not relevant?
Do you agree with Asher Peres that the Heisenberg Uncertainty Principle is an … ill-conceived notion … ?
Is your command of English language to fault for NOT replying to this question ?
Or you simply do NOT want to reply ? 🙂 🙂 🙂
Don’t worry : this is not a police interrogation : you are under NO obligation to reply …
As for being HONEST, well, this seems to be a less and less popular approach to more delicate issues even in science … 🙂 🙂 🙂
Dear Prof. Rosinger, maybe indeed some “lost in translation” with word “ill-conceived” happens, but I did answer your question and even pointed page number. I agree with existence of difficulties mentioned by Peres there.
By the way, another question, if you do not mind :
In a recently published paper, Prof. Hans de Raedt and collaborators did prove that the celebrated Bell Inequalities are IRRELEVANT in physics, since they are NOT violated either by classical, or by quantum systems. And the illusion that they would be violated by quantum systems is due to an elementary error in dealing with statistical data.
Details in this regard can be found in the recently published paper :
“The irrelevance of Bell inequalities in Physics : Comments on the DRHM paper”
http://hal.archives-ouvertes.fr/hal-00824124, \\ http://viXra.org/abs/1305.0129
Pingback: 20 years of qubits: the arXiv data | Quantum Frontiers