Author Archives: spiros

About spiros

Spyridon Michalakis does research on quantum many-body physics and topological quantum order at Caltech's Institute for Quantum Information and Matter, where he is also the manager for outreach activities.

Breaking up the band structure

Posted on by
1

Note from the editor: During the Summer of 2019, a group of thirteen undergraduate students from Caltech and universities around the world, spent 10 weeks on campus performing research in experimental quantum physics. Below, Aiden Cullo, a student from Binghampton University in New York, shares his experience working in Professor Yeh’s lab. The program, termed QuantumSURF, will run again during the Summer of 2020.

This summer, I worked in Nai-Chang Yeh’s experimental condensed matter lab. The aim of my project was to observe the effects of a magnetic field on our topological insulator (TI) sample, {(BiSb)}_2{Te}_3. The motivation behind this project was to examine more closely the transformation between a topological insulator and a state exhibiting the anomalous hall effect (AHE).

Both states of matter have garnered a good deal of interest in condensed matter research because of their interesting transport properties, among other things. TIs have gained popularity due to their applications in electronics (spintronics), superconductivity, and quantum computation. TIs are peculiar in that they simultaneously have insulating bulk states and conducting surface state. Due to time-reversal symmetry (TRS) and spin-momentum locking, these surface states have a very symmetric hourglass-like gapless energy band structure (Dirac cone).

The focus of our particular study was the effects of “c-plane” magnetization of our TI’s surface state. Theory predicts TRS and spin-momentum locking will be broken, resulting in a gapped spectrum with a single connection between the valence and conduction bands. This gapping has been theorized and shown experimentally in Chromium (Cr)-doped {(BiSb)}_2{Te}_3 and numerous other TIs with similar make-up.

In 2014, Nai-Chang Yeh’s group showed that Cr-doped {Bi}_2{Se}_3 exhibit this gap opening due to the surface state of {Bi}_2{Se}_3 interacting via the proximity effect with a ferromagnet. Our contention is that a similar material, Cr-doped {(BiSb)}_2{Te}_3, exhibits a similar effect, but more homogeneously because of reduced structural strain between atoms. Specifically, at temperatures below the Curie temperature (Tc), we expect to see a gap in the energy band and an overall increase in the gap magnitude. In short, the main goal of my summer project was to observe the gapping of our TI’s energy band.

Overall, my summer project entailed a combination of reading papers/textbooks and hands-on experimental work. It was difficult to understand fully the theory behind my project in such a short amount of time, but even with a cursory knowledge of topological insulators, I was able to provide a meaningful analysis/interpretation of our data.

Additionally, my experiment relied heavily on external factors such as our supplier for liquid helium, argon gas, etc. As a result, our progress was slowed if an order was delayed or not placed far enough in advance. Most of the issues we encountered were not related to the abstract theory of the materials/machinery, but rather problems with less complex mechanisms such as wiring, insulation, and temperature regulation.

While I expected to spend a good deal of time troubleshooting, I severely underestimated the amount of time that would be spent dealing with quotidian problems such as configuring software or etching STM tips. Working on a machine as powerful as an STM was frustrating at times, but also very rewarding as eventually we were able to collect a large amount of data on our samples.

An important (and extremely difficult) part of our analysis of STM data was whether patterns/features in our data set were artifacts or genuine phenomena, or a combination. I was fortunate enough to be surrounded by other researchers that helped me sift through the volumes of data and identify traits of our samples. Reflecting on my SURF, I believe it was a positive experience as it not only taught me a great deal about research, but also, more importantly, closely mimicked the experience of graduate school.

Ten finalists selected for film festival “Quantum Shorts”

Posted on by
Reply

“Crazy enough”, “visually very exciting”, “compelling from the start”, “beautiful cinematography”: this is what members of the Quantum Shorts festival shortlisting panel had to say about films selected for screening. As a member of the panel, and as someone who has experienced the power of visual storytelling firsthand (Anyone Can Quantum, Quantum Is Calling), I was excited to see filmmakers and students from around the world try their hand at interpreting the weirdness of the quantum realm in fresh ways.

The ten shortlisted films were chosen from a total of 203 submissions received during the festival’s 2016 call for entries. Some of the finalists are dramatic, some funny, some abstract. Some are live-action film, some animation. Each is under five minutes long. Find the titles and synopses of the shortlisted films below.

Screenings of the films start February 23 with confirmed events in Waterloo (23 February) and Vancouver (23 February), Canada; Singapore (25-28 February); Glasgow, UK (17 March); and Brisbane, Australia (24 March).

More details can be found at shorts.quantumlah.org, where viewers can also watch the films online
and vote for their favorite to help decide a ‘People’s Choice’ prize. The website also hosts interviews with the filmmakers.

The Quantum Shorts festival is run by the Centre for Quantum Technologies at the National University of Singapore with a constellation of prestigious partners including Scientific American magazine and the journal Nature. The festival’s media partners, scientific partners and screening partners span five countries. The Institute for Quantum Information and Matter at Caltech is a proud sponsor.

For making the shortlist, the filmmakers receive a $250 award, a one-year digital subscription to Scientific American and certificates.

The festival’s top prize of US $1500 and runner-up prize of US $1000 will now be decided by a panel of eminent judges. The additional People’s Choice prize of $500 will be decided by public vote on the shortlist, with voting open on the festival website until March 26th. Prizes will be announced by the end of March.

Quantum Shorts 2016: FINALISTS

Ampersand

What unites everything on Earth? That we are all ultimately composed of something that is both matter & wave

Submitted by Erin Shea, United States

Approaching Reality

Dancing cats, a watchful observer and a strange co-existence. It’s all you need to understand the essence of quantum mechanics

Submitted by Simone De Liberato, United Kingdom

Bolero

The coin is held fast, but is it heads or tails? As long as the fist remains closed, you are a winner – and a loser

Submitted by Ivan D’Antonio, Italy

Novae

What happens when a massive star reaches the end of its life? Something that goes way beyond the spectacular, according to this cosmic poem about the infinite beauty of a black hole’s birth

Submitted by Thomas Vanz, France

The Guardian

A quantum love triangle, where uncertainty is the only winner

Submitted by Chetan Kotabage, India

The Real Thing

Picking up a beverage shouldn’t be this hard. And it definitely shouldn’t take you through the multiverse…

Submitted by Adam Welch, United States

Together – Parallel Universe

It’s a tale as old as time: boy meets girl, girl is not as interested as boy hoped. So boy builds spaceship and travels through multi-dimensional reality to find the one universe where they can be together

Submitted by Michael Robertson, South Africa

Tom’s Breakfast

This is one of those days when Tom’s morning routine doesn’t go to plan – far from it, in fact. The only question is, can he be philosophical about it?

Submitted by Ben Garfield, United Kingdom

Triangulation

Only imagination can show us the hidden world inside of fundamental particles

Submitted by Vladimir Vlasenko, Ukraine

Whitecap

Dr. David Long has discovered how to turn matter into waveforms. So why shouldn’t he experiment with his own existence?

Submitted by Bernard Ong, United States

Greg Kuperberg’s calculus problem

Posted on by
8

“How good are you at calculus?”

This was the opening sentence of Greg Kuperberg’s Facebook status on July 4th, 2016.

“I have a joint paper (on isoperimetric inequalities in differential geometry) in which we need to know that

(\sin\theta)^3 xy + ((\cos\theta)^3 -3\cos\theta +2) (x+y) - (\sin\theta)^3-6\sin\theta -6\theta + 6\pi \\ \\- 6\arctan(x) +2x/(1+x^2) -6\arctan(y) +2y/(1+y^2)

is non-negative for x and y non-negative and 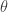\theta between 0 and \pi. Also, the minimum only occurs for x=y=1/(\tan(\theta/2).”

Let’s take a moment to appreciate the complexity of the mathematical statement above. It is a non-linear inequality in three variables, mixing trigonometry with algebra and throwing in some arc-tangents for good measure. Greg, continued:

“We proved it, but only with the aid of symbolic algebra to factor an algebraic variety into irreducible components. The human part of our proof is also not really a cake walk.

A simpler proof would be way cool.”

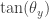I was hooked. The cubic terms looked a little intimidating, but if I converted x and y into \tan(\theta_x) and \tan(\theta_y), respectively, as one of the comments on Facebook promptly suggested, I could at least get rid of the annoying arc-tangents and then calculus and trigonometry would take me the rest of the way. Greg replied to my initial comment outlining a quick route to the proof: “Let me just caution that we found the problem unyielding.” Hmm… Then, Greg revealed that the paper containing the original proof was over three years old (had he been thinking about this since then? that’s what true love must be like.) Titled “The Cartan-Hadamard Conjecture and The Little Prince“, the above inequality makes its appearance as Lemma 7.1 on page 45 (of 63). To quote the paper: “Although the lemma is evident from contour plots, the authors found it surprisingly tricky to prove rigorously.”

As I filled pages of calculations and memorized every trigonometric identity known to man, I realized that Greg was right: the problem was highly intractable. The quick solution that was supposed to take me two to three days turned into two weeks of hell, until I decided to drop the original approach and stick to doing calculus with the known unknowns, x and y. The next week led me to a set of three non-linear equations mixing trigonometric functions with fourth powers of x and y, at which point I thought of giving up. I knew what I needed to do to finish the proof, but it looked freaking insane. Still, like the masochist that I am, I continued calculating away until my brain was mush. And then, yesterday, during a moment of clarity, I decided to go back to one of the three equations and rewrite it in a different way. That is when I noticed the error. I had solved for \cos\theta in terms of x and y, but I had made a mistake that had cost me 10 days of intense work with no end in sight. Once I found the mistake, the whole proof came together within about an hour. At that moment, I felt a mix of happiness (duh), but also sadness, as if someone I had grown fond of no longer had a reason to spend time with me and, at the same time, I had ran out of made-up reasons to hang out with them. But, yeah, I mostly felt happiness.

Greg Kuperberg pondering about the universe of mathematics.

Greg Kuperberg pondering about the universe of mathematics.

Before I present the proof below, I want to take a moment to say a few words about Greg, whom I consider to be the John Preskill of mathematics: a lodestar of sanity in a sea of hyperbole (to paraphrase Scott Aaronson). When I started grad school at UC Davis back in 2003, quantum information theory and quantum computing were becoming “a thing” among some of the top universities around the US. So, I went to several of the mathematics faculty in the department asking if there was a course on quantum information theory I could take. The answer was to “read Nielsen and Chuang and then go talk to Professor Kuperberg”. Being a foolish young man, I skipped the first part and went straight to Greg to ask him to teach me (and four other brave souls) quantum “stuff”. Greg obliged with a course on… quantum probability and quantum groups. Not what I had in mind. This guy was hardcore. Needless to say, the five brave souls taking the class (mostly fourth year graduate students and me, the noob) quickly became three, then two gluttons for punishment (the other masochist became one of my best friends in grad school). I could not drop the class, not because I had asked Greg to do this as a favor to me, but because I knew that I was in the presence of greatness (or maybe it was Stockholm syndrome). My goal then, as an aspiring mathematician, became to one day have a conversation with Greg where, for some brief moment, I would not sound stupid. A man of incredible intelligence, Greg is that rare individual whose character matches his intellect. Much like the anti-heroes portrayed by Humphrey Bogart in Casablanca and the Maltese Falcon, Greg keeps a low-profile, seems almost cynical at times, but in the end, he works harder than everyone else to help those in need. For example, on MathOverflow, a question and answer website for professional mathematicians around the world, Greg is listed as one of the top contributors of all time.

But, back to the problem. The past four weeks thinking about it have oscillated between phases of “this is the most fun I’ve had in years!” to “this is Greg’s way of telling me I should drop math and become a go-go dancer”. Now that the ordeal is over, I can confidently say that the problem is anything but “dull” (which is how Greg felt others on MathOverflow would perceive it, so he never posted it there). In fact, if I ever have to teach Calculus, I will subject my students to the step-by-step proof of this problem. OK, here is the proof. This one is for you Greg. Thanks for being such a great role model. Sorry I didn’t get to tell you until now. And you are right not to offer a “bounty” for the solution. The journey (more like, a trip to Mordor and back) was all the money.

The proof: The first thing to note (and if I had read Greg’s paper earlier than today, I would have known as much weeks ago) is that the following equality holds (which can be verified quickly by differentiating both sides):

4 x - 6\arctan(x) +2x/(1+x^2) = 4 \int_0^x \frac{s^4}{(1+s^2)^2} ds.

Using the above equality (and the equivalent one for y), we get:

F(\theta,x,y) = (\sin\theta)^3 xy + ((\cos\theta)^3 -3\cos\theta -2) (x+y) - (\sin\theta)^3-6\sin\theta -6\theta + 6\pi \\ \\4 \int_0^x \frac{s^4}{(1+s^2)^2} ds+4 \int_0^y \frac{s^4}{(1+s^2)^2} ds.

Now comes the fun part. We differentiate with respect to 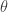\theta, x and y, and set to zero to find all the maxima and minima of F(\theta,x,y) (though we are only interested in the global minimum, which is supposed to be at x=y=\tan^{-1}(\theta/2)). Some high-school level calculus yields:

\partial_\theta F(\theta,x,y) = 0 \implies \sin^2(\theta) (\cos(\theta) xy + \sin(\theta)(x+y)) = \\ \\ 2 (1+\cos(\theta))+\sin^2(\theta)\cos(\theta).

At this point, the most well-known trigonometric identity of all time, 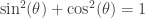\sin^2(\theta)+\cos^2(\theta)=1, can be used to show that the right-hand-side can be re-written as:

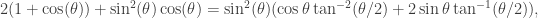
2(1+\cos(\theta))+\sin^2(\theta)\cos(\theta) = \sin^2(\theta) (\cos\theta \tan^{-2}(\theta/2) + 2\sin\theta \tan^{-1}(\theta/2)),

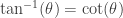where I used (my now favorite) trigonometric identity: \tan^{-1}(\theta/2) = (1+\cos\theta)/\sin(\theta) (note to the reader: \tan^{-1}(\theta) = \cot(\theta)). Putting it all together, we now have the very suggestive condition:

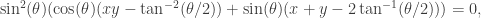
\sin^2(\theta) (\cos(\theta) (xy-\tan^{-2}(\theta/2)) + \sin(\theta)(x+y-2\tan^{-1}(\theta/2))) = 0,

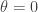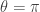noting that, despite appearances, \theta = 0 is not a solution (as can be checked from the original form of this equality, unless x and y are infinite, in which case the expression is clearly non-negative, as we show towards the end of this post). This leaves us with \theta = \pi and

\cos(\theta) (\tan^{-2}(\theta/2)-xy) = \sin(\theta)(x+y-2\tan^{-1}(\theta/2)),

as candidates for where the minimum may be. A quick check shows that:

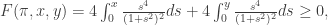
F(\pi,x,y) = 4 \int_0^x \frac{s^4}{(1+s^2)^2} ds+4 \int_0^y \frac{s^4}{(1+s^2)^2} ds \ge 0,

since x and y are non-negative. The following obvious substitution becomes our greatest ally for the rest of the proof:

x= \alpha \tan^{-1}(\theta/2), \, y = \beta \tan^{-1}(\theta/2).

Substituting the above in the remaining condition for \partial_\theta F(\theta,x,y) = 0, and using again that \tan^{-1}(\theta/2) = (1+\cos\theta)/\sin\theta, we get:

\cos\theta (1-\alpha\beta) = (1-\cos\theta) ((\alpha-1) + (\beta-1)),

which can be further simplified to (if you are paying attention to minus signs and don’t waste a week on a wild-goose chase like I did):

\cos\theta = \frac{1}{1-\beta}+\frac{1}{1-\alpha}.

As Greg loves to say, we are finally cooking with gas. Note that the expression is symmetric in \alpha and \beta, which should be obvious from the symmetry of F(\theta,x,y) in x and y. That observation will come in handy when we take derivatives with respect to x and y now. Factoring (\cos\theta)^3 -3\cos\theta -2 = - (1+\cos\theta)^2(2-\cos\theta), we get:

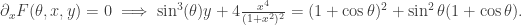
\partial_x F(\theta,x,y) = 0 \implies \sin^3(\theta) y + 4\frac{x^4}{(1+x^2)^2} = (1+\cos\theta)^2 + \sin^2\theta (1+\cos\theta).

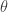Substituting x and y with \alpha \tan^{-1}(\theta/2), \beta \tan^{-1}(\theta/2), respectively and using the identities \tan^{-1}(\theta/2) = (1+\cos\theta)/\sin\theta and \tan^{-2}(\theta/2) = (1+\cos\theta)/(1-\cos\theta), the above expression simplifies significantly to the following expression:

4\alpha^4 =\left((\alpha^2-1)\cos\theta+\alpha^2+1\right)^2 \left(1 + (1-\beta)(1-\cos\theta)\right).

Using \cos\theta = \frac{1}{1-\beta}+\frac{1}{1-\alpha}, which we derived earlier by looking at the extrema of F(\theta,x,y) with respect to \theta, and noting that the global minimum would have to be an extremum with respect to all three variables, we get:

4\alpha^4 (1-\beta) = \alpha (\alpha-1) (1+\alpha + \alpha(1-\beta))^2,

where we used 1 + (1-\beta)(1-\cos\theta) = \alpha (1-\beta) (\alpha-1)^{-1} and

(\alpha^2-1)\cos\theta+\alpha^2+1 = (\alpha+1)((\alpha-1)\cos\theta+1)+\alpha(\alpha-1) = \\ (\alpha-1)(1-\beta)^{-1} (2\alpha + 1-\alpha\beta).

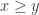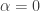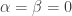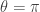We may assume, without loss of generality, that x \ge y. If \alpha = 0, then \alpha = \beta = 0, which leads to the contradiction \cos\theta = 2, unless the other condition, \theta = \pi, holds, which leads to 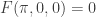F(\pi,0,0) = 0. Dividing through by \alpha and re-writing 4\alpha^3(1-\beta) = 4\alpha(1+\alpha)(\alpha-1)(1-\beta) + 4\alpha(1-\beta), yields:

4\alpha (1-\beta) = (\alpha-1) (1+\alpha - \alpha(1-\beta))^2 = (\alpha-1)(1+\alpha\beta)^2,

which can be further modified to:

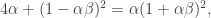
4\alpha +(1-\alpha\beta)^2 = \alpha (1+\alpha\beta)^2,

and, similarly for \beta (due to symmetry):

4\beta +(1-\alpha\beta)^2 = \beta (1+\alpha\beta)^2.

Subtracting the two equations from each other, we get:

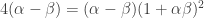4(\alpha-\beta) = (\alpha-\beta)(1+\alpha\beta)^2,

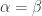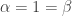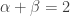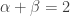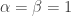which implies that \alpha = \beta and/or \alpha\beta =1. The first leads to 4\alpha (1-\alpha) = (\alpha-1)(1+\alpha^2)^2, which immediately implies \alpha = 1 = \beta (since the left and right side of the equality have opposite signs otherwise). The second one implies that either \alpha+\beta =2, or \cos\theta =1, which follows from the earlier equation \cos\theta (1-\alpha\beta) = (1-\cos\theta) ((\alpha-1) + (\beta-1)). If \alpha+\beta =2 and 1 = \alpha\beta, it is easy to see that \alpha=\beta=1 is the only solution by expanding (\sqrt{\alpha}-\sqrt{\beta})^2=0. 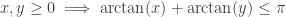If, on the other hand, \cos\theta = 1, then looking at the original form of F(\theta,x,y), we see that F(0,x,y) = 6\pi - 6\arctan(x) +2x/(1+x^2) -6\arctan(y) +2y/(1+y^2) \ge 0, since x,y \ge 0 \implies \arctan(x)+\arctan(y) \le \pi.

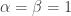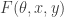And that concludes the proof, since the only cases for which all three conditions are met lead to \alpha = \beta = 1 and, hence, x=y=\tan^{-1}(\theta/2). The minimum of F(\theta, x,y) at these values is always zero. That’s right, all this work to end up with “nothing”. But, at least, the last four weeks have been anything but dull.

Update: Greg offered Lemma 7.4 from the same paper as another challenge (the sines, cosines and tangents are now transformed into hyperbolic trigonometric functions, with a few other changes, mostly in signs, thrown in there). This is a more hardcore-looking inequality, but the proof turns out to follow the steps of Lemma 7.1 almost identically. In particular, all the conditions for extrema are exactly the same, with the only difference being that cosine becomes hyperbolic cosine. It is an awesome exercise in calculus to check this for yourself. Do it. Unless you have something better to do.

Quantum Supremacy: The US gets serious

Posted on by
9

If you have been paying any attention to the news on quantum computing and the evolution of industrial and national efforts towards realizing a scalable, fault-tolerant quantum computer that can tackle problems intractable to current supercomputing capabilities, then you know that something big is stirring throughout the quantum world.

More than 15 years ago, Microsoft decided to jump into the quantum computing business betting big on topological quantum computing as the next big thing. The new website of Microsoft’s Station Q shows that keeping a low profile is no longer an option. This is a sentiment that Google clearly shared, when back in 2013, they decided to promote their new partnership with NASA Ames and D-Wave, known as the Quantum A.I. Lab, through a YouTube video that went viral (disclosure: they do own Youtube.) In fact, IQIM worked with Google at the time to get kids excited about the quantum world by developing qCraft, a mod introducing quantum physics into the world of Minecraft. Then, a few months ago, IBM unveiled the quantum experience website, which captured the public’s imagination by offering a do-it-yourself opportunity to run an algorithm on a 5-qubit quantum chip in the cloud.

But, looking at the opportunities for investment in academic groups working on quantum computing, companies like Microsoft were/are investing heavily in experimental labs across the pond, such as Leo Kowenhoven’s group at TU Delft and Charlie Marcus’ group in Copenhagen, with smaller investments here in the US. This may just reflect the fact that the best efforts to build topological qubits are in Europe, but it still begs the question why a fantastic idea like topologically protected majorana zero modes, by Yale University’s Nick Read and Dmitry Green, which inspired the now famous Majorana wire paper by Alexei Kitaev when he was a researcher at Microsoft’s Redmond research lab, and whose transition from theory to experiment took off with contributions from Maryland and IQIM researchers, was outsourced to European labs for experimental verification and further development. The one example of a large investment in a US academic research group has been Google’s hiring of John Martinis away from UCSB. In fact, John and I met a couple of years ago to discuss investment into his superconducting quantum computing efforts, because government funding for academic efforts to actually build a quantum computer was lacking. China was investing, Canada was investing, Europe went a little crazy, but the US was relying on visionary agencies like IARPA, DARPA and the NSF to foot the bill (without which Physics Frontiers Centers like IQIM wouldn’t be around). In short, there was no top-down policy directive to focus national attention and inter-agency Federal funding on winning the quantum supremacy race.

Until now.

The National Science and Technology Council, which is chaired by the President of the United States and “is the principal means within the executive branch to coordinate science and technology policy across the diverse entities that make up the Federal research and development enterprise”, just released the following report:

Advancing Quantum Information Science: National Challenges and Opportunities

The White House blog post does a good job at describing the high-level view of what the report is about and what the policy recommendations are. There is mention of quantum sensors and metrology, of the promise of quantum computing to material science and basic science, and they even go into the exciting connections between quantum error-correcting codes and emergent spacetime, by IQIM’s Pastawski, et al.

But the big news is that the report recommends significant and sustained investment in Quantum Information Science. The blog post reports that the administration intends “to engage academia, industry, and government in the upcoming months to … exchange views on key needs and opportunities, and consider how to maintain vibrant and robust national ecosystems for QIS research and development and for high-performance computing.”

Personally, I am excited to see how the fierce competition at the academic, industrial and now international level will lead to a race for quantum supremacy. The rivals are all worthy of respect, especially because they are vying for supremacy not just over each other, but over a problem so big and so interesting, that anyone’s success is everyone’s success. After all, anyone can quantum, and if things go according to plan, we will soon have the first generation of kids trained on hourofquantum.com (it doesn’t exist yet), as well as hourofcode.com. Until then, quantum chess and qCraft will have to do.

Remember to take it slow

Posted on by
3

“Spiros, can you explain to me this whole business about time being an illusion?”

These were William Shatner’s words to me, minutes after I walked into the green room at Silicon Valley’s Comic Con. The iconic Star Trek actor, best known for his portrayal of James Tiberius Kirk, captain of the starship Enterprise, was chatting with Andy Weir, author of The Martian, when I showed up at the door. I was obviously in the wrong room. I had been looking for the room reserved for science panelists, but had been sent up an elevator to the celebrity green room instead (a special room reserved for VIPs during their appearance at the convention). Realizing quickly that something was off, I did what anyone else would do in my position. I sat down. To my right was Mr. Weir and to my left was Mr. Shatner and his agent, Mr. Gary Hasson. For the first few minutes I was invisible, listening in casually as Mr. Weir revealed juicy details about his upcoming novel. And then, it happened. Mr. Shatner turned to me and asked: “And who are you?” Keep calm young man. You can outrun him if you have to. You are as entitled to the free croissants as any of them. “I am Spiros,” I replied. “And what do you do, Spiros?” he continued. “I am a quantum physicist at Caltech.” Drop the mic. Boom. Now I will see myself out before security…

comic_con_bg

“Spiros, can you explain to me this whole business about time being an illusion?”

Huh, I wonder if he means the… “You know, how there is no past, present or future in quantum mechanics,” Mr. Shatner continued. “Well, yes,” I responded, “that is called the arrow of time, an emergent direction in the time parameter found in the equation describing evolution in quantum physics. By the way, that time parameter itself is also emergent.” And then things got out of hand. “Wait a minute, are you telling me that not just the arrow of time, but time itself as a concept is an illusion?” asked Mr. Shatner with genuine excitement. “Yes. For starters, the arrow of time itself is a consequence of an emergent asymmetry between events that are all equally likely at the microscopic level. Think about flipping a fair coin one hundred times, for example. The probability of getting all heads is astronomically small. Zero point zero zero zero… with thirty zeroes before the one. Same is true if I ask you how likely it is that you flip fifty heads and then fifty tails,” I said and waited. “OK… still following,” Mr. Shatner assured me, so I continued, “but, say that you have trouble keeping track of all the different positions of the heads and tails; all you care about is counting how many times you flipped heads and how many times you flipped tails. What is the probability that you would count one hundred heads?” I asked. Mr. Shatner thought for a second, and so did Mr. Weir, before they answered almost in unison, “Well, it is still astronomically small. Just like before.” Yes! Holy cow, Batman, this is actually happening. I am having a conversation about physics with captain Kirk and the mastermind behind this year’s Golden Globe winner for Best Motion Picture: Musical or Comedy! This makes no sense! And I am not talking about the movie award – The Martian was hilarious.

andy-weir

“Exactly,” I replied. “But what about flipping the coin and counting fifty heads and fifty tails?” I asked. I could see that their wheels were spinning. What was I getting at? How was this different from before? “Does it have to be the first fifty heads, or can it be any which way, as long as it is fifty?” asked Mr. Weir. Bingo. “Any which way. We can only keep track of the number of them, not their position,” I reminded him. “Well, there are many more ways then to get fifty heads,” noted Mr. Shatner. “Yes there are,” I agreed and continued, “In fact, there are about one thousand billion billion billion combinations that all give fifty heads and fifty tails. In other words, one in ten times you flip a coin a hundred times, you will count exactly fifty heads and fifty tails. Think about this for a second. The probability of counting exactly fifty heads the first time you flip a coin a hundred times is thirty orders of magnitude larger than counting one hundred heads. Remember that any particular configuration of heads and tails is equally – astronomically – unlikely. But if you zoom out, then magic happens and an emergent asymmetry appears. A really huge asymmetry, at that.” They were hooked. It was time for the grand finale. “So, which events then are more likely for us to experience in the next second, if all of them are equally likely at some fundamental level?” I asked. Mr. Shatner responded first: “The ones that have billions of microscopic configurations that all look the same when you zoom out. Like the fifty heads thing.” Then, Mr. Weir, turning to Mr. Shatner added, “That’s the arrow of time following the direction of entropy as it increases.” I nodded (maybe a little too eagerly) and looked at my phone to see that it was close to noon. It would take me about five minutes to walk to Room 2 of the San Jose convention center, where Mr. Weir was to headline a panel titled “Let’s Go to Mars!” There was no way I was missing that panel. I knew that by now there would be a very long line of eager attendees waiting to hear Mr. Weir and Mr. Adam Savage (of Mythbusters fame) talk about Mars exploration. With some luck, I could walk there with Mr. Weir and sneak in without being noticed by the door police. I told Mr. Weir that it was time for us to go downstairs. He got up, I got up and…

“Spiros, where do you think you are going? Come here, sit right next to me. You promised to explain how time works. You can’t leave me hanging now!” Mr. Shatner was adamant.

I looked to Mr. Hasson and Mr. Weir, who were caught in the middle of this. “I… I can come back and we can talk more after Andy’s panel… My panel isn’t until 2 o’ clock,” I pleaded. Mr. Shatner did not think so. Science could not wait another second. He was actually interested in what I had to say, so I turned to Mr. Weir apologetically and he nodded with understanding and a “good luck, kid” kind-of-smile. Mr. Hasson seemed pleased with my choice and made some room for me to sit next to the captain.

william-shatner

“Now, where were we? Ah yes, you were going to explain to me how time itself is an illusion. Something about time in quantum evolution being emergent. What do you mean?” asked Mr. Shatner, cutting right to the chase. It was time for me to go all in: “Well, you see, there is this equation in quantum mechanics – Erwin Schrodinger came up with it – that tells us how the state of the universe at the quantum level changes with time. But where does time come from? Is it a fundamental concept, or is there something out there without which time itself cannot exist?” I waited for a second, as Mr. Shatner contemplated my question. He was stumped. What could possibly be more fundamental than time? Hmm… “Change,” I said. “Without change, there is no time and, thus, no quantum evolution. And without quantum evolution there is no classical evolution, no arrow of time. So everything hinges on the ability of the quantum state of the visible universe to change.” I paused to make sure he was following, then continued, “But if there is change, then where does it come from? Wherever it comes from, unless we end up with a timeless, unchanging and featureless entity, we will always be on the hook for explaining why it is changing, how it is changing and why it looks the way it does and not some other way,” I said and waited a second to let this sink in. “Spiros, if you are right, then how the heck can you get something out of nothing? If the whole thing is static, how come we are not frozen in time?” asked pointedly Mr. Shatner. “We are not the whole thing,” I said, maybe a bit too abruptly. “What do you mean we are not the whole thing? What else is there?” questioned Mr. Shatner. At this point I could see a large smile forming on Mr. Hasson’s face. His old friend, Bill Shatner, was having fun. A different kind of fun. A different kind of Comic Con. Sure, Bill still had to sit at a table in the main Exhibit Hall to greet thousands of fans, sign their favorite pictures of him and, for a premium, stand next to them for a picture that they would frame and display in their homes for decades to come. “Spiros, do you have a card?” interjected Mr. Hasson. Hmm, how do I say that this is not a thing among scientists… “I ran out. Sorry, everyone wants one these days, so… Here, I can type my email and number in your phone. Would that work?” I said, stretching the truth 1/slightly. “That would be great, thanks,” replied Mr. Hasson.

IMG_1168

With Mr. Stan Lee at the Silicon Valley Comic Con. At 93, Mr. Lee spent the whole weekend with fans, not once showing up at the green room to take a break. So I hunted him down with help from Mr. Hasson.

“Hey, stop distracting him! We are so close to the good stuff!” blasted Mr. Shatner. “Go on, now, Spiros. How does anything ever change?” asked Mr. Shatner with some urgency in his voice. “Dynamic equilibrium,” I replied. “Like a chemical reaction that is in equilibrium. You look from afar and see nothing happening. No bubbles, nothing. But zoom in a little and you see products and reactants dissolving and recombining like crazy, but always in perfect balance. The whole remains static, while the parts experience dramatic change.” I let this simmer for a moment. “We are not the whole. We are just a part of the whole. We are too big to see the quantum evolution as it happens in all its glory. But we are also too small to remain unchanged. Our visible universe is in dynamic equilibrium with a clock universe with which we are maximally entangled. We change only because the state of the clock universe changes randomly and we have no control over it, but to change along with it so that the whole remains unchanged,” I concluded, hoping that he would be convinced by a theory that had not seen the light of day until that fateful afternoon. He was not convinced yet. “Wait a minute, why would that clock universe change in the first place?” he asked suspiciously. “It doesn’t have to,” I replied, anticipating this excellent question, and went on, “It could remain in the same state for a million years. But we wouldn’t know it, because the state of our visible universe would have to remain in the same state also for a million years. We wouldn’t be able to tell that a million years passed between every microsecond of change, just like a person under anesthesia can’t tell that they are undergoing surgery for hours, only to wake up thinking it was just a moment earlier that they were counting down to zero.” He fell silent for a moment and then a big smile appeared on his face. “Spiros, you have an accent,” he said, as if stating the obvious. “Can I offer you a piece of advise?” he asked, in a calm voice. I nodded. “One day you will be in front of a large crowd talking about this stuff. When you are up there, make sure you talk slow so people can keep up. When you get excited, you start speaking faster and faster. Take breaks in-between,” he offered. I smiled and thanked him for the advise. By then, it was almost one o’ clock and Mr. Weir’s panel was about to end. I needed to go down there for real this time and meet up with my co-panelists, Shaun Maguire and Laetitia Garriott de Cayeux, since our panel was coming up next. I got up and as I was leaving the room, I heard from behind,

“Remember to take it slow, Spiros. When you are back, you will tell me all about how space is also an illusion.”

Aye aye captain!

Explaining Quantum Physics to Newton… in 140 characters

Posted on by
5

Sir Isaac Newton is considered by many as the greatest physicist of all time. But despite Newton’s contributions to classical physics, the man never even fathomed that the world was actually governed by the equations of quantum mechanics. We think it is time to right that wrong. With your help, we plan to send a Tweet back in time (something about time machines restricts quantum telegraphs to 140 characters) that explains what quantum is all about to Sir Isaac. This mini competition is taking place on our Twitter account over @IQIM_Caltech and ends on November 5th, 2015. The prize is a one-year digital subscription to Scientific American.

For more details, please see below. This mini competition is part of Quantum Shorts, a flash fiction competition conceived and executed by our friends at the Center for Quantum Technologies.

QSmini_oct28_twitter

Let the games begin!

Quantum Shorts 2015: A “flash fiction” competition

Posted on by
4

A blog on everything quantum is the perfect place to announce the launch of the 2015 Quantum Shorts competition. The contest encourages readers to create quantum-themed “flash fiction”: a short story of no more than 1000 words that is inspired by quantum physics. Scientific American, the longest continuously published magazine in the U.S., Nature, the world’s leading multidisciplinary science journal, and Tor Books, the leading science fiction and fantasy publisher, are media partners for the contest run by the Centre for Quantum Technologies at the National University of Singapore. Entries can be submitted now through 11:59:59 PM ET on December 1, 2015 at http://shorts.quantumlah.org.

“Quantum physics seems to inspire creative minds, so we can’t wait to see what this year’s contest will bring,” says Scientific American Editor in Chief and competition judge Mariette DiChristina.

A panel of judges will select the winners and runner-ups in two categories: Open and Youth. The public will also vote and decide the People’s Choice Prize from entries shortlisted across both categories. Winners will receive a trophy, a cash prize and a one-year digital subscription to ScientificAmerican.com. The winner of the Open category will also be featured on ScientificAmerican.com.

QS2015_bannerThe quantum world offers lots of scope for enthralling characters and mind-blowing plot twists, according to Artur Ekert, director of the Centre for Quantum Technologies and co-inventor of quantum cryptography. “A writer has plenty to play with when science allows things to be in two places – or even two universes – at once,” he says. “The result might be funny, tense or even confusing. But it certainly won’t be boring.” Artur is one of the Open category judges.

Another judge is Colin Sullivan, editor of Futures, Nature’s own science-themed fiction strand. “Science fiction is a powerful and innovative genre,” Colin says. “We are excited to see what kinds of stories quantum physics can inspire.”

The 2015 Quantum Shorts contest is also supported by scientific partners around the world. The Institute for Quantum Information and Matter is proud to sponsor this competition, along with our friends at the Centre for Engineered Quantum Systems, an Australian Research Council Centre of Excellence, the Institute for Quantum Computing at the University of Waterloo, and the Joint Quantum Institute, a research partnership between the University of Maryland and the National Institute of Standards and Technology.

Submissions to Quantum Shorts 2015 are limited to 1000 words and can be entered into the Quantum Shorts competition via the website at http://shorts.quantumlah.org, which also features a full set of rules and guidelines.

For more information about the organizer and partners, please visit the competition website at http://shorts.quantumlah.org.

The mentors that shape us

Posted on by
12

Three years and three weeks ago I started my first blog. I wasn’t quite sure what to call it, so I went to John for advice. I had several names in mind, but John quickly zeroed in on one: Quantum Frontiers. The url was available, the name was simple and to the point, it had the word quantum in it, and it was appropriate for a blog that was to provide a vantage point from which the public could view the frontiers of quantum science. But there was a problem; we had no followers and when I first asked John if he would write something for the blog, he had said: I don’t know… I will see…maybe some day… let me think about it. The next day John uploaded More to come, the first real post on Quantum Frontiers after the introductory Hello quantum world! We had agreed on a system in order to keep the quality of the posts above some basic level: we would send each other our posts for editing before we made them public. That way, we could catch any silly typos and have a second pair of eyes do some fact-checking. So, when John sent me his first post, I went to task editing away typos. But the power that comes with being editor-in-chief corrupts. So, when I saw the following sentence in More to come…

I was in awe of Wheeler. Some students thought he sucked.

I immediately changed it to…

I was in awe of Wheeler. Some students thought less of him.

And next, when I saw John write about himself,

Though I’m 59, few students seemed awed. Some thought I sucked. Maybe I did sometimes.

I massaged it into…

Though I’m 59, few students seemed awed. Some thought I was not as good. Maybe I wasn’t sometimes.

When John published the post, I read it again for any typos I might have missed. There were no typos. I felt useful! But when I saw that all mentions of sucked had been restored to their rightful place, I felt like an idiot. John did not fire a strongly-worded email back my way asking for an explanation as to my taking liberties with his own writing. He simply trusted that I would get the message in the comfort of my own awkwardness. It worked beautifully. John had set the tone for Quantum Frontier’s authentic voice with his very first post. It was to be personal, even if the subject matter was as scientifically hardcore as it got.

So when the time came for me to write my first post, I made it personal. I wrote about my time in Los Alamos as a postdoc, working on a problem in mathematical physics that almost broke me. It was Matt Hastings, an intellectual tornado, that helped me through these hard times. As my mentor, he didn’t say things like Well done! Great progress! Good job, Spiro! He said, You can do this. And when I finally did it, when I finally solved that damn problem, Matt came back to me and said: Beyond some typos, I cannot find any mistakes. Good job, Spiro. And it meant the world to me. The sleepless nights, the lonely days up in the Pajarito mountains of New Mexico, the times I had resolved to go work for my younger brother as a waiter in his first restaurant… those were the times that I had come upon a fork on the road and my mentor had helped me choose the path less traveled.

When the time came for me to write my next post, I ended by offering two problems for the readers to solve, with the following text as motivation:

This post is supposed to be an introduction to the insanely beautiful world of problem solving. It is not a world ruled by Kings and Queens. It is a world where commoners like you and me can become masters of their domain and even build an empire.

Doi-Inthananon-Thailand

Doi-Inthananon temple in Chiang Mai, Thailand. A breathtaking city, host of this year’s international math olympiad.

It has been way too long since my last “problem solving” post, so I leave you with a problem from this year’s International Math Olympiad, which took place in gorgeous Chiang Mai, Thailand. FiverThirtyEight‘s recent article about the dominance of the US math olympic team in this year’s competition, gives some context about the degree of difficulty of this problem:

Determine all triples (a, b, c) of positive integers such that each of the numbers: ab-c, bc-a, ca-b is a power of two.

Like Fermat’s Last Theorem, this problem is easy to describe and hard to solve. Only 5 percent of the competitors got full marks on this question, and nearly half (44 percent) got no points at all.

But, on the triumphant U.S. squad, four of the six team members nailed it.

In other words, only 1 in 20 kids in the competition solved this problem correctly and about half of the kids didn’t even know where to begin. For more perspective, each national team is comprised of the top 6 math prodigies in that country. In China, that means 6 out of something like 100 million kids. And only 3-4 of these kids solved the problem.

The coach of the US national team, Po-Shen Loh, a Caltech alum and an associate professor of mathematics at Carnegie Mellon University (give him tenure already) deserves some serious props. If you think this problem is too hard, I have this to say to you: Yes, it is. But, who cares? You can do this.

Note: I will work out the solution in detail in an upcoming post, unless one of you solves it in the comments section before then!

Update: Solution posted in comments below (in response to Anthony’s comment). Thank you all who posted some of the answers below. The solution is far from trivial, but I still wonder if an elegant solution exists that gives all four triples. Maybe the best solution is geometric? I hope one of you geniuses can figure that out!

Ant-Man and the Quantum Realm

Posted on by
12

ant-man-2015-marvel-movieIt was the first week of August last summer and I was at LAX for a trip to North Carolina as a guest speaker at Project Scientist’s STEM summer camp for young women. I had booked an early morning flight and had arrived at my gate with time to spare, so I decided to get some breakfast. I walked by a smart-looking salad bar and thought: Today is the day. Moving past the salad bar, I ordered a juicy cheeseburger with fries at the adjacent McDonald’s. Growing up in Greece, eating McDonald’s was a rare treat; as was playing video games with my brothers and reading comic books late at night. Yet, through a weird twist of fate, it was these last two guilty pleasures that were to become my Hadouken!, my Hulk, Smash! of choice for breaking down the barriers between the world of quantum mechanics and the everyday reality of our super-normal, super-predictable lives.

I finished my burger, stuffing the last few delicious fries in my mouth, when my phone buzzed – I had a call from The Science and Entertainment Exchange, a non-profit organization funded by the National Academy of Sciences, whose purpose is to bring leading scientists in contact with Hollywood in order to elevate the level of science in the movies. I was to report to Atlanta, GA for a movie consult on a new superhero movie: Ant-Man. As I listened to the director of the Exchange discuss the assignment, I grumbled to myself: Why can’t I be the guy who works on Thor? Who is this Ant-Man anyways? But, in typical Hollywood fashion, the call had a happy ending: “Paul Rudd is Ant-Man. He may be at the meeting, but I can’t promise anything.” Marvel would cover my expenses, so my reluctant reply went something like this:

Hell yeah.

The meeting was in three days time. I would finish my visit to Queens University in Charlotte, NC and take the next flight out to Atlanta. But first, some fun and games were in order. As part of my visit to Project Scientist’s camp, I was invited to teach quantum mechanics to a group of forty girls, ages 8-12, all of whom were interested in science, engineering and mathematics and many of whom did not have the financial means to pursue these interests outside of the classroom. So, I went to Queens University with several copies of MinecraftEDU, the educational component to one of the most popular video games of all time: Minecraft. As I described in “Can a game teach kids quantum mechanics”, I spent the summer of 2013 designing qCraft, a modification (mod) to Minecraft that allows players to craft blocks imbued with quantum superpowers such as quantum superposition and quantum entanglement. The mod, developed in collaboration with Google and TeachersGaming, became popular, amassing millions of downloads around the world. But it is one thing to look at statistics as a measure of success, and another to look into the eyes of these girls as they lost themselves in a game (qCraft is free to download and comes with an accompanying curriculum) designed to teach them the heady concepts that inspired Richard Feynman to quip: If you think you understand quantum theory, you don’t.

girls_qcraftMy visit to Charlotte was so wonderful that I nearly decided to cancel my trip to Atlanta in order to stay with the girls and their mentors until the end of the week. But Mr. Rudd deserved the very best science could offer, so I boarded my flight and resolved to bring Project Scientist to Caltech the next summer. On my way to Atlanta, I used the in-flight WiFi to do some research on Ant-Man. He was part of the original Avengers, a founding member, in fact (nice!) His name was Dr. Hank Pym. He had developed a particle, aptly named after himself, which allowed him to shrink the space between atoms (well now…) He embedded vials of that particle in a suit that allowed him to shrink to the size of an ant (of course, that makes sense.) In short, he was a mad, mad scientist. And I was called in to help his successor, Scott Lang (Paul Rudd’s character), navigate his way through the physics of shrinking. Holy guacamole Ant-man! How does one shrink the space between atoms? As James Kakalios, author of The Physics of Superheroes, puts it in a recent article on Nate Silver’s FiveThirtyEight:

We’re made of atoms, and the neighboring atoms are all touching each other. One method of changing your size that’s out: Just squeeze the atoms closer together.
…
So the other option: What determines the size of atoms anyway?
…
We can calculate this with quantum mechanics, and it turns out to be the ratio of fundamental constants: Planck’s constant and the mass of an electron and the charge of the electron and this and that. The thing that all these constants have in common is that they’re constant. They don’t change.

Wonderful. Just peachy. How am I supposed to come up with a way that will allow Ant-Man to shrink to the size of an ant, if one of the top experts in movie science magic thinks that our best bet is to somehow change fundamental constants of nature?

Enter Pinewood Studios

The flight was longer than I expected, which gave me time to think. A limo was waiting for me at the airport; I was to be taken directly to Pinewood Studios, luggage in hand and all. Once I was at my destination, I was escorted to the 3rd floor of a nondescript building, accessible only through special authorization (nice touch, Marvel). I was shown to what seemed like the main conference room, an open area with a large conference table. I expected that I would have to wait an hour before anyone showed up, so I started fiddling with my phone, making myself look busy. The next time I looked up, Paul Rudd was tapping my shoulder, dressed in sweats after what seemed like a training session for The 300.

The Meeting

Within minutes, the director (Peyton Reed), the writers, producers, VFX specialists, computer playback experts (I became friends with their supervisor, Mr. Matthew Morrissey, who went to great lengths to represent the atoms on-screen as clouds of probability, in the s, p, d, f orbital configurations you see flashing in quantum superposition behind Hank Pym at his lab) and everyone else involved with the movie was in the room. I sat at the head of the long table with Paul next to me. He asked most of the questions along with the director, but at the time I didn’t know Paul was involved with writing the script. We discussed a lot of things, but what got everyone excited was when I told them that the laws of physics as we know them may break down as we delve deeper and deeper into the quantum realm. You see, all of the other superheroes, no matter how strong and super, had powers that conformed to the laws of physics (stretching them from time to time, but never breaking them). But if someone could go to a place where the laws of physics as we know them were not yet formed, at a place where the arrow of time was broken and the fabric of space was not yet woven, the powers of such a master of the quantum realm would only be constrained by their ability to come back to the same (or similar) reality from which they departed. All the superheroes of Marvel and DC Comics combined would stand no chance against Ant-Man with a malfunctioning regulator…

The Quantum Realm

The birth of the term itself is an interesting story. Brad Winderbaum, co-producer for the movie, emailed me a couple of days after the meeting with the following request: Could I come up with a term describing Ant-Man going to the “microverse”? The term “microverse” carried legal baggage, so something fresh was needed. I offered “going nano”, “going quantum”, “going atomic”, or… “quantum realm”. I didn’t know how small the “microverse” scale was supposed to be in a writer’s mind (in a physicist’s mind it is exactly 10^{-6} meters – one thousandth of a millimeter), hence the many options. The reply was quick:

Thanks Spiros! Quantum Realm is a pretty great term.

Et voilà. Ant-Man was going to the quantum realm, a place where time and space dissolve and the only thing strong enough to anchor Scott Lang to reality is… You have to watch the movie to see what that is – it was an off-the-cuff remark I made at the meeting… At the end of the meeting, Paul, Peyton and the others thanked me and asked me if I could fly to San Francisco the next week for the first week of shooting. There, I would have met Michael Douglas and Evangeline Lilly, but I declined the generous offer. It was the week of Innoworks Academy at Caltech, an award-winning summer camp for middle school kids on the free/reduced lunch program. As the camp’s adviser, I resolved to never miss a camp as long as I was in the country and San Francisco is in the same state as Caltech. My mom would be proud of my decision (I hope), though an autograph from Mr. Douglas would have fetched me a really good hug.

The Movie

I just watched the movie yesterday (it is actually good!) and the feeling was surreal. Because I had no idea what to expect. Because I never thought that the people in that room would take what I was saying seriously enough to use it in the movie. I never got a copy of the script and during the official premiere three weeks ago, I was delivering a lecture on the future of quantum computing in a monastery in Madrid, Spain. When I found out that Kevin Feige, president of Marvel Studios, said this at a recent interview, my heart skipped several beats:

But the truth is, there is so much in Ant-Man: introducing a new hero, introducing a very important part of technology in the Marvel universe, the Pym particles. Ant-Man getting on the Avengers’ radar in this film and even – this is the weirdest part, you shouldn’t really talk about it because it won’t be apparent for years – but the whole notion of the quantum realm and the whole notion of going to places that are so out there, they are almost mind-bendingly hard to fathom. It all plays into Phase Three.

The third phase of the Marvel Cinematic Universe is about to go quantum and all I can think of is: I better start reading comic books again. But first, I have to teach a bunch of 8-12 year-old girls quantum physics through Minecraft. It is, after all, the final week of Project Scientist here at Caltech this summer and the theme is coding. With quantum computers at the near horizon, these young women need to learn how to program Asimov’s laws of quantum robotics into our benevolent quantum A.I. overlords. These young women are humanity’s new hope…

Apply to join IQIM!

Posted on by
Reply

Editor’s Note: Dr. Chandni Usha is an IQIM postdoctoral scholar working with Prof. Eisenstein. We asked her to describe her experience as an IQIM fellow.

Just another day at work!

Just another day at work!

When I look back at how I ended up here, I find myself in a couple of metastable states. Every state pushed me to newer avenues of knowledge. Interestingly, growing up I never really knew what it was like to be a scientist. I had not watched any of those sci-fi movies or related TV series as a kid. No outreach program ever reached me in my years of schooling! My first career choice was to be a lawyer. But a casual comment by a friend that lawyers are ‘liars’ was strong enough to change my mind. Strangely enough, now the quest is for the truth, in a lab down at the sub-basement of one of the world’s best research institutes.

I did my masters in Physics at this beautiful place called the Indian Institute of Science in Bangalore. I realized that I like doing things with my hands. Fixing broken instruments seemed fun. Every new data point on a plot amused me. It was more than obvious that experimental physics is where my heart was and hence I went on to do a Ph.D. in condensed matter physics. When I decided to apply for postdoctoral positions, an old friend of mine, Debaleena Nandi, told me to look up the IQIM website. That was in November 2012, and I applied for the IQIM postdoctoral fellowship. My stars were probably aligned to be here. Coincidentally, Jim Eisenstein, my adviser, was in India on a sabbatical and I happened to hear him give a talk. It left such a strong impression in my mind that I was willing to give up on a trip to Europe for an interview the next day had he offered me a position. We spoke about possible problems, but no offer was in sight and hence I did travel to Europe with my mind already at Caltech. IQIM saved me from my dilemma when they offered me the fellowship a few days later.

Now, why choose IQIM! Reason number one was Jim. And reason number two was this blog which brought in this feeling that there exists a community here; where experimentalists and theorists could share their ideas and grow together in a symbiotic manner. My first project was with an earlier postdoc, Erik Henriksen, who is now a faculty at Washington University in St. Louis. It was based on a proposal by fellow IQIM professor Jason Alicea which involved decorating a film of graphene with a certain heavy metal adatom. Jason’s prediction was that if you choose the right adatom, it could endow some of its unique properties such as strong spin-orbit coupling to the underlying graphene sheet. One can thus engineer graphene to what is called a topological insulator where only the edges of the graphene sheet conduct. Erik had taken on this task and I tagged along. Working in a very small campus with a close-knit community helps bounce your ideas around others and that’s how this experiment came into being. I found it particularly interesting that Jason and his colleagues often ask us, the experimentalists, whether some of the ideas they have are actually feasible to be performed in a lab!

The IQIM fellowship allows you to work on a variety of fields that come under the common theme of quantum information and matter. In addition to providing an independent funding and research grant, the fellowship offers the flexibility to work with any mentor and even multiple mentors, especially in the theory group. In experimental groups however, that flexibility is limited but not impossible. The fellowship gives you a lot of freedom and encourages collaborations. IQIM theory folks have a very strong and friendly group with a lot of collaborations, to the extent that it is often hard to distinguish the faculty from the postdocs and students.

Apart from a yearly retreat to a beautiful resort in Lake Arrowhead, the social life at IQIM is further enhanced through the Friday seminars where you get to hear about the work from postdocs and graduate students from IQIM, as well as other universities. IQIM’s outreach activities have been outstanding. A quick look at this blog will take you from the PhD Comics animations, to teaching kids quantum mechanics through Miinecraft, to hosting middle school students at the InnoWorks academy and a host of other activities. This note will not be complete without mentioning about our repeated efforts to attract women candidates. My husband lives in India, and I live right across the globe, all for the love of science. I am not alone in this respect as we have two more women postdocs at IQIM who have similar stories to tell. So, if you are a woman and wish to pursue a quality research program, this is the place to be, for together we can bring change.

Now that I have convinced you that IQIM is something not to be missed, kindly spread the word. And if you are looking for an awesome opportunity to work at Caltech, get your CV and research statement and apply for the fellowships before Dec 5, 2014!